15. ÖMG-Kongress
Jahrestagung der Deutschen Mathematikervereinigung

16. bis 22. September 2001 in Wien

Sektion 12 - Wahrscheinlichkeitstheorie, Statistik
Dienstag, 18. September 2001, 19.00, Hörsaal 7

 

Calculating the Modes of a Multinomial Distribution

Ulrich Dieter, TU Graz

 

 

The probability of the event $ (k_1, k_2, ,... k_r)$ of the multinomial distribution is given by the formula

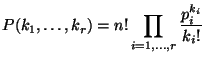$\displaystyle P(k_1, \dots, k_r) = n! \prod_{i=1,\dots,r} \frac{p_i^{k_i}}{k_i!}$ (1)

where

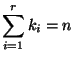$\displaystyle \sum_{i=1}^r k_i = n$   and$\displaystyle \quad \sum_{i=1}^r p_i = 1. $

The task of this paper is to find all maxima of (1), usually called the modes of the multinomial distribution.

Two methods for this exist. One is based on Moran's inequality, which is not presented in its sharper version in textbooks like Feller [1957] or Johnson- Kotz-Balakrishnan [1997]. It is slow for large values of the number $ r$ of the $ p_i$. By far more effective is the idea of Finucan [1964] to consider the step-function

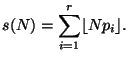$\displaystyle s(N) = \sum_{i=1}^r \lfloor N p_i \rfloor.$ (2)

The jumps of $ s(N)$ can be determined quite easily. If $ s(N)=n$ holds, $ k_i=\lfloor N p_i \rfloor$ are the components of the unique mode. If there are more than one mode their components $ k_i$ can be determined as $ k_i=\lfloor N p_i \rfloor$ or $ k_i=\lfloor N p_i \rfloor -1$. The subscripts $ i$ where $ 1$ has to be subtracted are easy to determine. Numerical examples are given which exhibit the method.

By a similar method the modes of a hypergeometric distribution can be calculated.

[1] W. Feller [1968], An Introduction to Probability Theory and its Applications. J. Wiley 1950, 1957 and 1968.
[2] H.M. Finucan [1964], The mode of a Multinomial Distribution. Biometrica 51, 513-517.
[3] N.L. Johnson [1997], S. Kotz and N. Balkrishnan, Discrete Multivariate Distributions. J. Wiley 1997.

Zeitplan der Sektion   Tagesübersicht   Liste der Vortragenden